Solve Pythagorean Identities Calc
 Pythagorean Identities
Pythagorean Identities
An online pythagorean identities solver.
Pythagorean identities
| a) | sin2θ + cos2θ | = | 1. |
| b) | 1 + tan2θ | = | sec2θ |
| c) | 1 + cot2θ | = | csc 2θ |
Proof of the Pythagorean identities
Proof 1. According to the Pythagorean theorem,
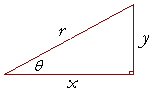
x2 + y2 = r2. . . . . . . . . . . . . . . .(1)
Therefore, on dividing both sides by r2,
| x2 r2 |
+ | y2 r2 |
= | r2 r2 |
= 1. |
cos2θ + sin2θ = 1. . . . . . . . . . . . . .(2)
Apart from the order of the terms, this is the first Pythagorean identity, a)
To derive b), divide line (1) by x2; to derive c), divide by y2.
Or, we can derive both b) and c) from a) by dividing it first by cos2θ and then by sin2θ. On dividing line 2) by cos2θ, we have
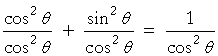
That is,
1 + tan2θ = sec2θ.
And if we divide a) by sin2θ, we have
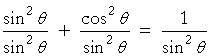
That is,
1 + cot2θ = csc2θ.
The 3 Pythagorean identities are thus equivalent to one another.
Proof 2.

| sin2θ + cos2θ | = | y2 r2 |
+ | x2 r2 |
| = | y2 + x2 r2 |
= | r2 r2 |
|
| According to the Pythagorean theorem, = 1 | ||||
 Similar Algebra Calculator ►
Similar Algebra Calculator ►
Adding Complex Number Calculator
Subtracting Complex Number Calculator
Polynomial Equation Calculator
Multiplying Complex Number Calculator
Angle Between Vectors Calculator
3D & 2D Vector Magnitude Calculator
Polynomial Addition Calculator